Rolling element bearings are manufactured in a variety of formats. Below you will find a procedure for selecting the correct rolling element bearing type. From this the correct size can be selected.

Friction in rolling element: A rough and ready rule
Friction in rolling element bearings generates heat which can eventually destroy the bearing. With friction in mind, a common rule of thumb used for the allowable speed of ball and straight roller bearings is:

( B + D ) · n/2 < 500,000
Where	B	= bore diameter in millimeters
D		= outside diameter in millimeters
n		= speed in rpm

Selection of an antifriction bearing for a particular application:
We now look at one method for selecting a rolling element bearing given a load/life specification. The following table gives a qualitative overview of the characteristics of each rolling element bearing type. We use this table to select the configuration of the bearing.

Bearing Type	Direction of Load			Ratio of Load			Misalignment Capacity
	radial	axial	both	high	med	low	high	med	low
Thrust Ball		y			y				y
Deep Groove Ball	y		y		y			y
Cylindrical Roller	y		certain types		y				y
Needle Roller	y			y					y
Tapered Roller	y	y	y		y				y
Self-aligning Ball	y		y			y	y
Self-aligning Spherical Roller	y		y		y		y
Angular Contact Ball		y	y			y			y

Now that we have decided upon the bearing type, we can move on to the more quantitative issue of sizing the bearing. Two metrics that are needed for bearing specification are the static and dynamic load capacities. Static load capacity can specify the bearing if rotational speed is slow, intermittent, and/or subject to shocks. Dynamic load capacity is used when the bearing rotational speed is smooth and relatively constant.

Static Load Specification:
The axial and radial forces acting on the stationary rotary bearing determine the Basic Static Load Rating listed in bearing catalogs. When there are both axial and radial loads on a bearing, the combined static load can be found as follows.

Fstatic = Xsrad · Fsrad + Xsax · Fsax
If only radial forces act,
Fstatic = Fsrad
Where	Fstatic	= The combined, equivalent static bearing load
Fsrad		= The static radial load
Fsax		= The static axial load
Xsrad		= The static radial factor (dimensionless)
Xsax		= The static axial factor (dimensionless)

Values of So depend upon the requirements for low-noise operation and the type of bearing, as shown in the following table.

If the bearing is stationary for extended periods or rotates slowly and/or intermittently and is subject to shock loads, then the selection is based upon this basic load rating. Values of basic load rating, Co, for each bearing are quoted in the bearing catalogs.

Dynamic Load Specification:
The dynamic load specification of a rotary bearing is dependent on both the dynamic and static forces acting upon the bearing. Therefore, please first calculate the Static Load Specification as outlined above. Axial and radial static forces multiplied by dynamic factors combine to form the equivalent dynamic bearing load, which is calculated as follows.

Fdyn = Xdrad · Fsrad + Xdax · Fsax
Where	Fdyn	= Equivalent dynamic bearing load
Fsrad		= Static radial load on bearing
Fsax		= static axial load on bearing
Xdrad		= radial dynamic factor (dimensionless)
Xdax		= axial dynamic factor (dimensionless)

When F_sax = 0 or is relatively small up to F_sax/F_dyn = e (The values of F_srad, F_sax, and e are given in the rolling element bearing data) then

Since we have calculated the equivalent dynamic bearing load we can now compute the bearing dynamic load rating, which is used to select the bearing. Catalog dynamic load rating values should be chosen higher than the computed value for safety.

The catalog-listed dynamic load ratings are dependent upon both the equivalent dynamic load and the required bearing life. The ISO equation for the basic rating life is:

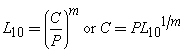
Where	L =	basic rated life (millions of revolutions)
C =		basic dynamic load rating
P =		equivalent dynamic bearing load
m =		exponent in the life equation, m = 3 for ball bearings m = 3.3 for other bearings.

Basic Rated Life of Bearings:
The basic rated life is defined as the number of revolutions that ninety percent of a group of identical bearings would be expected to achieve. It is determined via the life required of the bearing. Typical life requirements for various machine categories are listed below.

Machine Usage Type	Life Required of Bearings (Hours)
household appliances — intermittent use	300 - 3000
hand tools, construction equipment — short period use	3000 - 8000
lifts, cranes — high reliability for short periods	8000 - 12000
8h/day gears, motors — full day partial use	10000 - 25000
8h/day machine tools, fans — full day full use	20000 - 30000
continuous use	40000 - 50000